MP Board Class 11th Maths Important Questions Chapter 1 Sets
Sets Important Questions
Sets Objective Type Questions
(A) Choose the correct option:
Question 1.
A set is defind as:
(a) Collection of an object
(b) Collection of well defind object
(c) Nothing can be said
(d) None of these.
Answer:
(b) Collection of well defind object
Question 2.
If A = {1, 2, 3},B = {2, 3, 4} and U = {1, 2, 3, 4, 5, 6},then A’ ∩ B’ =
(a) {2, 3}
(b) {1, 5,6}
(c) {5, 6}
(d) {1, 4, 5, 6}.
Answer:
(c) {5, 6}
Question 3.
A and B are two sets, then A ∩ (A ∪ B) =
(a) A
(b) B
(c) ϕ
(d) None of these.
Answer:
(a) A
![]()
Question 4.
A set A = {x : x ∈ R, x2 = 16 and 2x = 6} =
(a) ϕ
(b) {14,3,4}
(c) {3}
(d) {4}.
Answer:
(a) ϕ
Question 5.
For a non – empty set A:
(a) A∪A’ = A
(b) A∪A’ = A’
(c) A∪A’ = U
(d) A∪A’ = ϕ .
Answer:
(c) A∪A’ = U
Question 6.
If two non – empty sets A and B are not equal, then:
(a) A⊂A∩B
(b) B⊂A∩B
(c) A∩B⊂B
(d) A∩B⊂ϕ.
Answer:
(c) A∩B⊂B
Question 7.
A and B are two sets and n(A) = 70, n(B) = 60 and n(A∪B) = 110, then n(A∩B) =
(a) 240
(b) 50
(c) 40
(d) 20.
Answer:
(d) 20.
Question 8.
If A = {1,2,3,4,5}, then the number of proper subsets:
(a) 120
(b) 30
(c) 31
(d) 32.
Answer:
(d) 32.
Question 9.
If A, B and C are three sets, then A – (B∪C) is equal to:
(a) (B – A)∩C
(b) (A – B) ∪C
(c) (A – B) ∩(A – C)
(d) (A – B) ∪(A – C)
Answer:
(c) (A – B) ∩(A – C)
![]()
Question 10.
Let S = {1,2,3,4}. The total numbers of unordered pair of disjoint subsets of S is equal to:
(a) 25
(b) 34
(c) 42
(d) 41.
Answer:
(d) 41.
(B) Match the following:
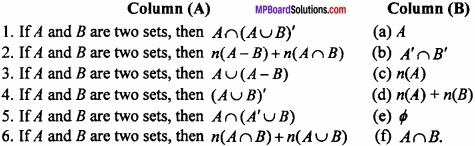
Answer:
- (e)
- (c)
- (a)
- (b)
- (f)
- (d)
(C) Fill in the blanks:
- If the elements of A mid B are 3 and 6, then minimum number of elements in A∪B is …………………………….
- If X and Y are two sets such that n(X) = 17, n(Y) = 23 and n(X∪Y) = 38, then n(X∩Y) = …………………………
- If sets A = {1, 2, 3, 4, 5, 6} and B = {2, 4, 6, 8}, then B – A = ……………………………..
- A set which does not contain any element is called ………………………….
- If set A has n elements, then the number of subsets of A are …………………………..
- If A = {1, 2} and B = {3, 4}, then (A∪B)∩ϕ = ……………………………..
Answer:
- 6
- 2
- {8}
- Empty set
- 2n
- ϕ
(D) Write true/false:
- If A ∩ B = B, then B ⊂ A.
- If A = {1, 2, 3, 4} and B = {2, 3, 4, 5, 6}, then A ∆ B = {1, 5, 6}.
- If n(A – B) = n(A) – n(A n B).
- For a non – empty sets A is A∩A’- A’.
- If A = {1, 2, 3, 4}, then n[P(A)] = 16.
Answer:
- False
- True
- True
- False
- True.
![]()
Question (E)
Write answer in one word/sentence:
- If A = {1, 2}, then write all the subsets of set A.
- If A = {4, 5, 8, 12} and B = {1, 4, 6, 9}, then find the value of A – (B – A).
- If n(U) = 700, n(A) = 200, n(B) = 300, and n(A∩B) = 100, then find the value of n(A’∩B’). ‘
- Find the value of n[P{P{P(ϕ}}]
- If A = {a, b, c, d}, then find all subsets of set A.
- If U = {1, 2, 3, 4, 5, 6, 7, 8, 9}, B = {2, 4, 6, 8} and C = {3, 4, 5, 6}, then find the value of (B – C)’.
Answer:
- ϕ, {1}, {2}, {1,2}
- {4, 5, 8, 12}
- 300
- 4
- 16
- {1, 3, 4, 5, 6, 7, 8, 9}.
Sets Very Short Answer Type Questions
Question 1.
Write the following sets in set builder form: (NCERT)
- {3, 6, 9, 12}
- {2, 4, 8, 16, 32}
- {5, 25, 125, 625}
- {2, 4, 6,},
- {1, 4, 9, ………… 100}.
Solution:
- A = {x : x is a natural number, multiple of 3 and x < 15}
- B = {x : x = 2n, x∈N and n < 6}
- C = {x : x = 5n, and x∈N and n ≤ 4}
- D = {x : x is an even natural number}
- E = {x : x = n2, x ∈ N and n < 11}.
Question 2.
Write the following sets in roster form: (NCERT)
- A = {x : x is an odd natural number}
- B = {x : x is an integer – \(\frac{1}{2}\) < x < \(\frac{9}{2}\)
- C = {x : x is an integer x2 ≤ 4}
- D = {x : x is a letter of a word LOYAL}
- E = {x : x is a month of year which does not have 31 days}
- F – {x : x is a consonant of English alphabet which comes before k}
Solution:
- A = {1, 3, 5, 7, 9}
- B = {0, 1, 2, 3, 4}
- C = {-2, -1, 0, 1, 2}
- D = {L, 0, Y, A}
- E = {FEBRUARY, APRIL, JUNE, SEPTEMBER, NOVEMBER}
- F = {b, c, d, f, g, h, j}.
![]()
Question 3.
From following find whether A = B. (NCERT)
- A = {a, b, c, d}, B = {d, c, b, a}.
- A = {4, 8, 12, 16}, B = {8,4, 16, 18}.
- A = {2, 4, 6, 8, 10}, B = {x : x is a positive even integer x < 10}.
- A = {x : x is a multiple of 10}, B = {10, 15, 20, 25, 30}.
Solution:
1. A = {a, b, c, d}, B = {d, c, b, a}
All the elements of set A is in set B
A = B.
2. A = {4, 8, 12, 16}, B = {8, 4, 16, 18}
All the elements of A is not in set B.
∴ A≠B.
3. Here A = {2, 4, 6, 8, 10} and B = { x : x is an even number and x ≤ 10}
B = {2, 4, 6, 8, 10}
i.e., The elements of set A and B are same.
∴ A = B
4. Here A = {x : x is a multiple of 10} = {10, 20, 30, 40}
and B = {10, 15, 20, 25, 30, …}
The elements of set A and B are not same.
∴ A≠B.
Question 4.
If A = {1, 2, 3, 4}, B = {3, 4, 5, 6}, C = {5, 6, 7, 8} and D = {7, 8, 9, 10} then find the following: (NCERT)
- A∪B
- A∪C
- B∪C
- B∪D
Solution:
- A ∪ B = {1, 2, 3, 4} ∪ {3, 4, 5, 6} = {1, 2, 3, 4, 5, 6}.
- A ∪ C = {1, 2, 3, 4} ∪ {5, 6, 7, 8} = {1, 2, 3, 4, 5, 6, 7, 8}.
- B ∪ C = {3, 4, 5, 6} ∪ {5, 6, 7, 8} = {3, 4, 5, 6, 7, 8}
- B ∪ D = {3, 4, 5, 6} ∪ {7, 8, 9, 10} = {3, 4, 5, 6, 7, 8, 9, 10}
![]()
Question 5.
If A = {3, 5, 7, 9, 11}, B = {7, 9, 11, 13}, C= {11, 13, 15} and D = {15, 17} then, find the following:
- A ∩ B
- B ∩ C
- A ∩ C
- B ∩ D
Solution:
- A ∩ B = {3, 5, 7, 9, 11} ∩ {7, 9, 11, 13} = {7, 9, 11}
- B ∩ C = {7, 9, 11, 13} ∩ {11, 13, 15} = {11, 13}
- A ∩ C = {3, 5, 7, 9, 11} ∩ {11, 13, 15} = {11}
- B ∩ D = {7, 9, 11, 13} ∩ {15, 17} = ϕ
Question 6.
If A = {3, 6, 9, 12, 15, 18, 21}, B = {4, 8, 12, 16, 20}, C = {2, 4, 6, 8, 10, 12, 14, 16} and D = {5, 10, 15, 20} then, find the following: (NCERT)
- A – B
- A – C
- A – D
- B – A
- C – A
- D – A
- B – C
- B – D
- C – B
- D – B
- C – D
- D – C?
Solution:
Let A and B are two sets and A – B is a set of those elements which one present in A and not in B.
1. A – B = {Those elements present in A and not in B}
= {3, 6, 9, 12, 15, 18, 21} – {4, 8, 12, 16, 20}
= {3, 6, 9, 15, 18, 21}.
2. A – C = {Those elements present in A and not in C}
= {3, 6, 9, 12, 15, 18, 21} – {2, 4, 6, 8, 10, 12, 14, 16}
= {3, 9, 15, 18, 21}.
3. A – D = {The elements which are in A but not in D}
= {3, 6, 9, 12, 15, 18, 21} – {5, 10, 15, 20}
= {3, 6, 9, 12, 18, 21}.
4. B – A = {The elements which are in B but not in A}
= {4, 8, 12, 16, 20} – {3, 6, 9, 12, 15, 18, 21}
= {4, 8, 16, 20}.
5. C – A = {The elements which are in C but not in A}
= {2, 4, 6, 8, 10, 12, 14, 16} – {3, 6, 9, 12, 15, 18, 21}
= {2, 4, 8, 10, 14, 16}.
6. D – A = {The elements which are in D but not in A}
= {5, 10, 15, 20} – {3, 6,9, 12, 15, 18, 21}
= {5, 10, 20}.
7. B – C = {The elements which are in B but not in C}
= {4, 8, 12, 16, 20} – {2, 4,6, 8, 10, 12, 14, 16}
= {20}.
8. B – D = {The elements which are in B but not in D}
= {4, 8, 12, 16, 20} – {5, 10, 15, 20}
= {4, 8, 12, 16}.
9. C – B = {The elements which are in C but not in B}
= {2, 4, 6, 8, 10, 12, 14, 16} – {4, 8, 12, 16, 20}
= {2, 6, 10, 14}.
10. D – B = {The elements which are in D but not in B}
= {5, 10, 15,20} – {4, 8, 12, 16, 20}
= {5, 10, 15}.
11. C – D = {The elements which are in C but not in D}
= {2, 4, 6, 8, 10, 12, 14, 16} – {5, 10, 15, 20}
= {2, 4, 6, 8, 12, 14, 16}.
12. D – C = {The elements which are in D but not in C}
= {5, 10, 15, 20} – {2, 4, 6, 8, 10, 12, 14, 16}
= {5, 15, 20}.
![]()
Question 7.
Let U = {1, 2, 3, 4, 5, 6, 7, 8, 9}, A = {1, 2, 3, 4}, B = {2, 4, 6, 8} and C then find the following:
- A’
- B’
- (A ∪ C)’
- (A ∩ B)’
- (A’)’
- (B – C)’
- (B – C)’
Solution:
1. A’= U – A
= {1, 2, 3, 4, 5, 6, 7, 8, 9} – {1, 2, 3, 4}
⇒ A’= {5, 6, 7, 8, 9}.
2. B’ = U – B
⇒ {1, 2, 3, 4, 5, 6, 7, 8, 9} – {2, 4, 6, 8}
⇒ B’ = {1, 3, 5, 7, 9}.
3. (A ∪ C) = {1, 2, 3, 4} ∪ {3, 4, 5, 6}
⇒ A ∪ C = {1, 2, 3, 4, 5, 6}
(A ∪ C)’= U – (A ∪ C)
= {1, 2, 3, 4, 5, 6, 7, 8, 9} – {1, 2, 3, 4, 5, 6}
⇒ (A ∪ C)’ = {7, 8, 9}
4. A ∪ B = {1, 2, 3, 4} ∪ {2, 4, 6, 8} = {1, 2, 3, 4, 6, 8}
(A ∪ B)’ = ∪ – (A ∪ B)
= {1, 2, 3, 4, 5, 6, 7, 8, 9} – {1, 2, 3, 4, 6, 8}
⇒ (A ∪ B)’ = {5, 7, 9}
5. (A’)’ = ∪ – A’
= {1, 2, 3, 4, 5, 6, 7, 8, 9} – {5, 6, 7, 8, 9}
⇒ (A’)’= {1, 2, 3, 4} = A
6. B – C = {2, 4, 6, 8} – {3, 4, 5, 6} = {2, 8}.
(B ∪ C)’ = U – (B – C)
= {1, 2, 3, 4, 5, 6, 7, 8, 9} – {2, 8}
⇒ (B – C)’= {1, 3, 4, 5, 6, 7, 9}.
Sets Short Answer Type Questions
Question 1.
If X and Fare two sets, n(X) = 17, n(Y) = 23 and n(X ∪ F) = 38, then find n(X ∩ F)? (NCERT)
Solution:
We know that n(X ∪ Y) = n(X) + n(Y) – n(X∩Y)
Given: n(X) = 17, n(Y) = 23, n(X ∪ Y) = 38, n(X ∩ Y) = ?
∴ 38 = 17 + 23 – n(X ∩ Y)
⇒ 38 = 40 – n(X ∩ Y)
⇒ n(X ∩ Y) = 40 – 38
⇒ n(X ∩ Y) = 2.
![]()
Question 2.
If X and Y are two sets, such that X∪Y has 18 elements, X has 8 elements and F has 15 elements, then how many elements does X ∩ Y have? (NCERT)
Solution:
Given: n(X ∪ Y) = 18, n(X) = 8, n(Y) = 15.
Applying formula n(X ∪ Y) = n(X) + n(Y) – n(X ∩ Y)
18 = 8 + 15 – n(X ∩ Y)
⇒ n(X ∩ Y) = 23 – 18 = 5.
Question 3.
If S and Tare two sets, such that S has 21 elements, T has 32 elements and S∩T has 11 elements, how many elements does S∪T have? (NCERT)
Solution:
Given: n(S) = 21, n(T) = 32, n(S ∩ T) = 11, n(S ∪ T) = ?
∵ n(S ∪ T) = n(S) + n(T) – n(S ∩ T)
⇒ n(S ∪ T) = 21 + 32 – 11 = 42.
![]()
Question 4.
If X and Y are two sets such that X has 40 elements, X∪Y has 60 elements and X∩Y has 10 elements, then how many elements does Y have? (NCERT)
Solution:
Given: n(X) = 40, n(X ∪ Y) = 60, n(X ∩ Y) = 10, n(Y) = ?
Applying formula n(X ∪ Y) = n(X) + n(Y) – n{X ∩ Y)
⇒ 60 = 40 + n(Y) – 10
⇒ 60 = 30 + n(Y)
⇒ n(Y) = 30.
Question 5.
If A = {1, 2, 3, 4}, B = {3, 4, 5, 6}, C = {5, 6, 7, 8} and D = {7, 8, 9, 10}, then find the following: (NCERT)
- A ∪ B ∪ C
- A ∪ B ∪ D
- B ∪ C ∪ D.
Solution:
1. A ∪ B ∪ C = {1, 2, 3, 4} ∪ {3, 4, 5, 6} ∪ {5, 6, 7, 8}
= {1, 2, 3, 4, 5, 6} ∪ {5, 6, 7, 8}
= {1, 2, 3, 4, 5, 6, 7, 8}.
2. A ∪ B ∪ D = { 1, 2, 3, 4} ∪ {3, 4, 5, 6} ∪{7, 8, 9, 10}
= {1, 2, 3, 4, 5, 6} ∪ {7, 8, 9, 10}
= {1, 2, 3, 4, 5, 6, 7, 8, 9, 10}.
3. B ∪ C ∪ D = {3, 4, 5, 6} ∪ {5, 6, 7, 8} ∪ {7, 8, 9, 10}
= {3, 4, 5, 6} ∪ {5, 6, 7, 8, 9, 10}
= {3, 4, 5, 6, 7, 8, 9, 10}.
Sets Long Answer Type Questions
Question 1.
In a survey of 600 students in a school, 150 students were found to be taking tea and 225 taking coffee, 100 were taking both tea and coffee. Find how many students were taking neither tea nor coffee? (NCERT)
Solution:
Let C and T be the students taking coffee and tea.
Here, n(T) = 150, n(C) = 225, n(C ∩ T) = 100.
Applying formula n(C ∪ T) = n(T) + n(C) – n(C ∩ T)
n(C ∪ T) = 150 + 225 – 100 = 375 – 100
⇒ n(C ∪ T) = 275
Total number of students = 600 = n(U).
Number of students taking neither tea nor coffee = n(C ∪ T)’
n(C ∪ T)’ = n(U) – n(C ∪ T)
= 600 – 275 = 325.
![]()
Question 2.
In a group of 70 people, 37 likes coffee, 52 likes tea and each person likes at least one of these two drinks. Find the number of persons who likes both coffee and tea? (NCERT)
Solution:
Let C and T be the persons who likes coffee and tea.
Given: n(C) = 37, n(T) = 52, n(C ∪ T) = 70, n(C ∩ T) = ?
Applying formula n(C ∪ T) = n(C) + n(T) – n(C ∩ T)
⇒ 70 = 37 + 52 – n(C ∩ T)
⇒ 70 = 89 – n(C ∩ T)
⇒ n(C ∩ T) = 89 – 70
⇒ n(C ∩ T) = 19
∴ Number of people who likes both coffee and tea = 19.
Question 3.
In a group of 65 people, 40 likes cricket, 10 likes both cricket and tennis. How many like tennis only and not cricket? How many like tennis? (NCERT)
Solution:
Let C and T denotes the people who likes cricket and tennis respectively.
Given: n(C ∪ T) = 65, n(C) = 40, n(C ∩ T) = 10
We know that
n(C ∪ T) = n(C) + n(T) – (C ∩ T)
65 = 40 + n(T) – 10
⇒ n(T) = 65 – 30 = 35.
∴ Number of people who likes only tennis and not cricket
= n(T ∩ C)’ = n(T) – (C ∩ T)
= 35 – 10 = 25.
Question 4.
In a survey of 60 people, it was found that 25 people read newspaper H, 26 read newspaper T, 26 read newspaper I, 9 read both H and I. 11 read both H and T, 8 read both T and 1.3 read all three newspapers. Find
- The number of people who read at least one of the newspapers.
- The number of people who read exactly one newspaper. (NCERT)
Solution:
Given: n (H) = 25, n (T) = 26, n(I) = 26, n(H ∩ I) = 9, n(H ∩ T) = 11, n(T ∩ I) = 8, n(H ∩ T ∩ I) = 3
1. The number of people who reads at least one of the newspaper = n(H∪T∪I)
= n(H) + n(T) + n(I) – n(H ∩ T) – n(T ∩ I) – n(H ∩ I) + n(H ∩ T ∩ I)
= 25 + 26 + 26 – 11 – 8 – 9 + 3
= 77 – 28 + 3 = 80 – 28 = 52.
2. The number of people who reads exactly one newspaper.
= n(H) + n(T) + n(I) – 2n(H ∩ I) – 2n(H ∩ T) – 2n(T ∩ I) + 3n(H ∩ T ∩ I)
= 25 + 26 + 26 – 2 × 9 – 2 × 11 – 2 × 8 + 3 × 3 = 77 – 18 – 22 – 16 + 9
= 86 – 56 = 30.
![]()
Question 5.
In a survey it was found that 21 people liked product A, 26 liked product B and 29 liked product C. If 14 people liked products A and B, 12 people liked products C and A, 14 people liked products B and C and 8 liked all the three products. Find how many liked product C only? (NCERT)
Solution:
Let A, B and C denotes the people liked the products A, B and C respectively.
Given: n(A) = 21, n(B) = 26, n(C) = 29, n(A ∩ B) = 14, n(C ∩ A) = 12, n(B ∩ C) = 14, n(A ∩ B ∩ C) = 8
n(only C) = n(C) – n(C ∩ A) – n(B ∩ C) + n(A ∩ B ∩ C)
= 29 – 12 – 14 + 8
= 37 – 26 = 11.