MP Board Class 8th Maths Solutions Chapter 1 Rational Numbers Ex 1.1
Mp Board Class 8 Maths Chapter 1 Question 1.
Using appropriate properties, find.

Solution:
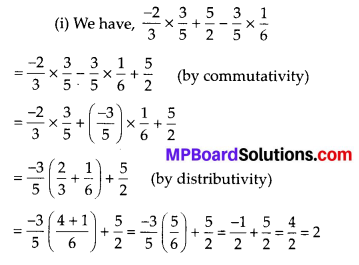
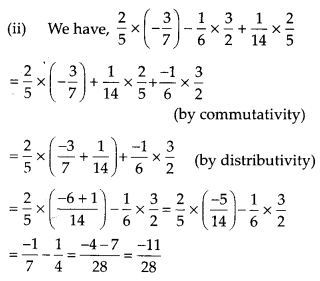
Mp Board Class 8th Maths Chapter 1 Question 2.
Write the additive inverse of each of the following

Solution:

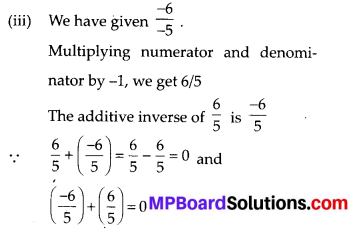
(iv) We have given \(\frac{2}{-9}\)
Multiplying numerator and denominator by -1, we get \(\frac{2}{-9}\)
The additive inverse of \(\frac{2}{-9}\) is \(\frac{2}{9}\)
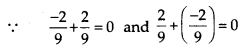
(v) We have \(\frac{19}{-6}\)
Multiplying numerator and denominator by -1, we get \(\frac{-19}{6}\)
![]()
![]()
Class 8 Maths Chapter 1 Mp Board Question 3.
Verify that -(-x) = x for
(i) x = \(\frac{11}{15}\)
(ii) x = \(-\frac{13}{17}\)
Solution:

Mp Board Solution Class 8 Maths Chapter 1 Question 4.
Find the multiplicative inverse of the following.

Solution:
(i) We have given, -13
The multiplicative inverse of -13 is \(\left(\frac{-1}{13}\right)\)
![]()
(ii) We have given, \(\frac{-13}{19}\)
The multiplicative inverse of is \(\frac{-13}{19}\) is \(\frac{-19}{13}\)
![]()
(iii) We have given, \(\frac{1}{5}\)
The multiplicative inverse of \(\frac{1}{5}\) is 5.
∵ \(\frac{1}{5} \times 5=1\)
(iv) We have given, \(\frac{-5}{8} \times \frac{-3}{7}\)
The multiplicative inverse of

(v) We have given, \(-1 \times \frac{-2}{5}\)
The multiplicative inverse of

(vi) We have given, -1.
The multiplicative inverse of -1 is -1.
∵ (-1) × (-1) = 1.
Mp Board Class 8 Maths Solutions English Medium Question 5.
Name the property under multiplication used in each of the following.
Solution:
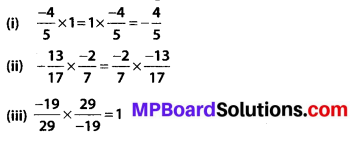
(i) We have given, \(\frac{-4}{5} \times 1=1 \times \frac{-4}{5}=\frac{-4}{5}\)
i.e., 1 is the multiplicative identity. Thus, it is a identity property under multiplication
(ii) We have given, \(\frac{-13}{17} \times \frac{-2}{7}=\frac{-2}{7} \times \frac{-13}{17}\), which shows the commutativity.
Thus, it is a commutative property under multiplication.
(iii) We have given \(\frac{-19}{29} \times \frac{29}{-19}=1\), which shows that \(\frac{29}{-19}\) is a multiplicative inverse of \(\left(\frac{-19}{29}\right)\)
Thus, it is a inverse property under multiplication.
![]()
Mp Board Class 8th Maths Solutions English Medium Question 6.
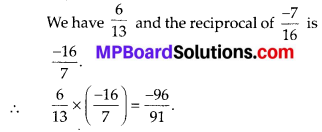
Multiply \(\frac{6}{13}\) by the reciprocal of \(\frac{-7}{16}\).
Solution:
Maths Class 8 Mp Board English Medium Solution Question 7.
Tell what property allows you to compute \(\frac{1}{3} \times\left(6 \times \frac{4}{3}\right) \text { as }\left(\frac{1}{3} \times 6\right) \times \frac{4}{3}\)
Solution:
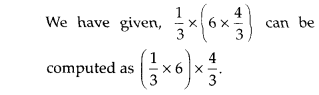
Above property is associativity.
[ ∵ a × (b × c) = (a × b) × c]
![]()
Mp Board Solution Class 8 Maths Question 8.
Is \(\frac{8}{9}\) the multiplicative inverse of \(-1 \frac{1}{8}\) ? Why or why not?
Solution:
We have given a fraction \(\frac{8}{9}\) and \(-1 \frac{1}{8}=\frac{-9}{8}\)
No, \(\frac{-9}{8}\) is not a multiplicative inverse of \(\frac{8}{9}\) because \(\frac{8}{9} \times\left(\frac{-9}{8}\right)=-1 \neq 1\)
Mp Board Solution Class 8 Math Question 9.
Is 0.3 the multiplicative inverse of \(3 \frac{1}{3}\) ? Why or why not?
Solution:

![]()
Mp Board 8th Class Maths Book Solutions Question 10.
Write.
(i) The rational number that does not have a reciprocal.
(ii) The rational numbers that are equal to their reciprocals.
(iii) The rational number that is equal to its negative.
Solution:
(i) 0 is the rational number, which does not have a reciprocal.
(ii) 1 and (-1) are the rational numbers, that are equal to their reciprocals.
(iii) 0 is the rational number that is equal to its negative.
![]()
Class 8 Mp Board Maths Solution Question 11.
Fill in the blanks.
(i) Zero has …… reciprocal.
(ii) The numbers …… and ……. are their own reciprocals.
(iii) The reciprocals of -5 is ……
(iv) Reciprocal of \(\frac{1}{x}\), where x ≠ 0 is ……
(v) The product of two rational numbers is always a ……
(vi) The reciprocal of positive rational number is …….
Solution:
(i) Zero has no reciprocal.
(ii) The numbers 1 and -1 are their own reciprocals.
(iii) The reciprocal of -5 is \(\frac{-1}{5}\) .
(iv) Reciprocal of \(\frac{1}{x}\), where x ≠ 0 is x.
(v) The product of two rational numbers is always a rational number.
(vi) The reciprocal of positive rational number is positive.