In this article, we share MP Board Class 12th Maths Book Solutions Chapter 2 प्रतिलोम त्रिकोणमितीय फलन Ex 2.2 Pdf, These solutions are solved by subject experts from the latest MP Board books.
MP Board Class 12th Maths Book Solutions Chapter 2 प्रतिलोम त्रिकोणमितीय फलन Ex 2.2
निम्नलिखित को सिद्ध कीजिए-
प्रश्न 1.
3 sin-1 x = sin-1(3x – 4x3), x ϵ \(\left[-\frac{1}{2}, \frac{1}{2}\right]\)
हल:
माना sin-1x = y
⇒ x = sin y
R.H.S. = sin-1 (3x – 4x3)
= sin-1(3 sin y – 4 sin3 y)
= sin-1 (sin 3y)
(∵ sin 3y = 3 sin y – 4 sin3y)
= 3y (∵ sin-1 sin x = x)
= 3 sin-1 x
= L.H.S.
अत: 3 sin-1x = sin-1(3x – 4x3)
प्रश्न 2.
3 cos-1 x = cos-1 (4x3 – 3x), x ϵ \(\left[\frac{1}{2}, 1\right]\)
हल:
सिद्ध करना है
3cos-1 x = cos-1 (4x3 – 3x) …(1)
माना cos-1 = y
⇒ x = cosy
∴ समी० (1) का RH.S.
=cos-1(4x3 – 3x)
= cos-1 (4cos3 y – 3cos y)
=cos-1 (cos 3y)
= 3y
= 3cos-1x (∵ cos-1 x = y)
अत: 3cos-1 = cos-1 (4x3 – 3x)
प्रश्न 3.
tan-1\(\frac{2}{11}\) + tan-1\(\frac{7}{24}\) = tan-1\(\frac{1}{2}\)
हल:
हम जानते हैं कि
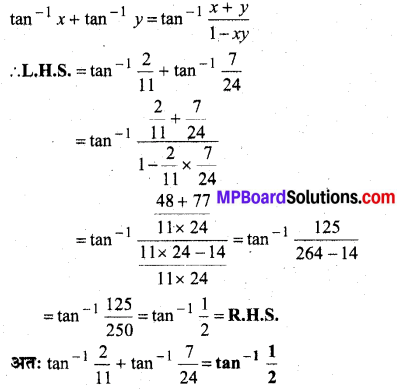
प्रश्न 4.
2 tan-1\(\frac{1}{2}\) + tan-1\(\frac{1}{7}\) = tan-1\(\frac{31}{17}\)
हल:
2 tan-1\(\frac{1}{2}\) + tan-1\(\frac{1}{7}\) = tan-1\(\frac{31}{17}\)
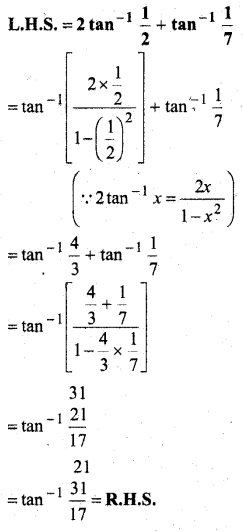
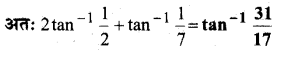
निम्नलिखित फलनों को सरलतम रूप में लिखिए-
प्रश्न 5.
tan-1 \(\frac{\sqrt{1+x^{2}}-1}{x}\), x ≠ 0
हल:
माना x = tan θ ⇒ θ = tan-1x

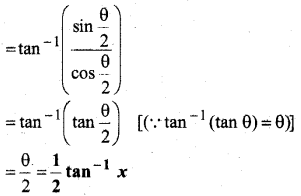
प्रश्न 6.
tan-1\(\frac{1}{\sqrt{x^{2}-1}}\), |x| > 1.
हल:
माना x = secθ ⇒ θ = sec-1x
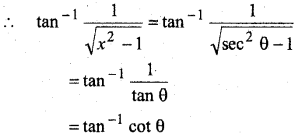

![]()
प्रश्न 7.
tan-1\((\sqrt{\frac{1-\cos x}{1+\cos x}})\), 0 < x < π
हल:
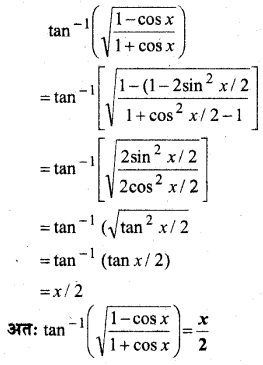
प्रश्न 8.
tan-1\(\left(\frac{\cos x-\sin x}{\cos x+\sin x}\right), \frac{-\pi}{4}<x<\frac{3 \pi}{4}\)
हल:

प्रश्न 9.
tan-1\(\frac{x}{\sqrt{a^{2}-x^{2}}}\), |x| < a
हल:
माना x = a sin θ ⇒ \(\frac{x}{a}\) = sin θ
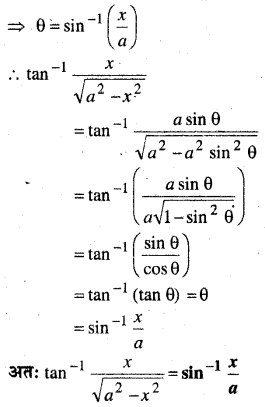
प्रश्न 10.

हल:
माना x = a tan θ ⇒ \(\frac{x}{a}\) = tan θ
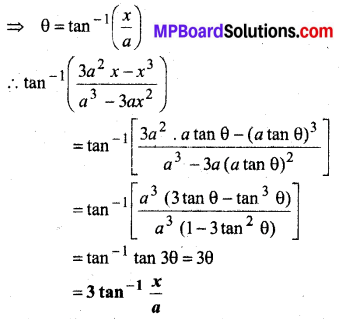
निम्नलिखित में से प्रत्येक का मान ज्ञात कीजिए
प्रश्न 11.
tan-1[2cos (2sin-1\(\frac{1}{2}\))]
हल:
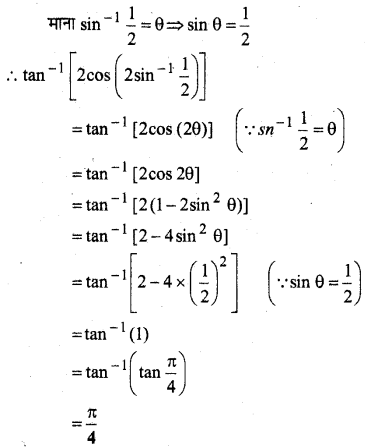
![]()
प्रश्न 12.
cot (tan-1 a + cot-1 a)
हल:
माना y = cot (tan-1a + cot-1a)
= cot\(\frac{\pi}{2}\) [∵ tan-1a + cot-1a = \(\frac{\pi}{2}\)]
= 0 [∵ cot \(\frac{\pi}{2}\) = 0]
प्रश्न 13.
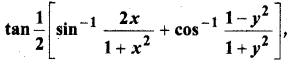
|x| < 1, y > 0 तथा xy < 1
हल:
माना
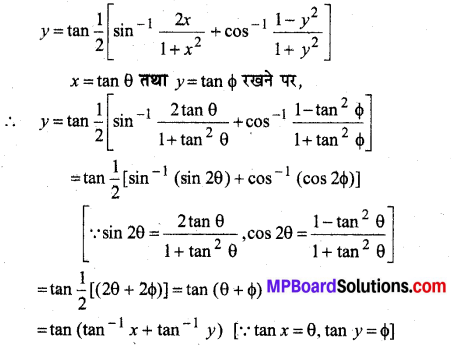
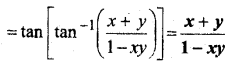
प्रश्न 14.
यदि sin(sin-1\(\frac{1}{5}\) + cos-1x) = 1, तो x का मान ज्ञात कीजिए।
हल:
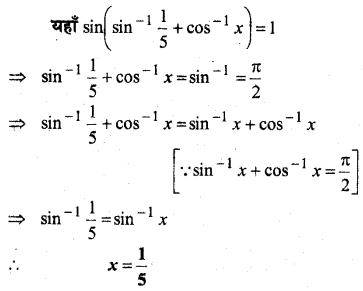
प्रश्न 15.
यदि tan-1 \(\frac{x-1}{x-2}\) + tan-1\(\frac{x+1}{x+2}=\frac{\pi}{4}\), तो x का मान ज्ञात कीजिए।
हल:
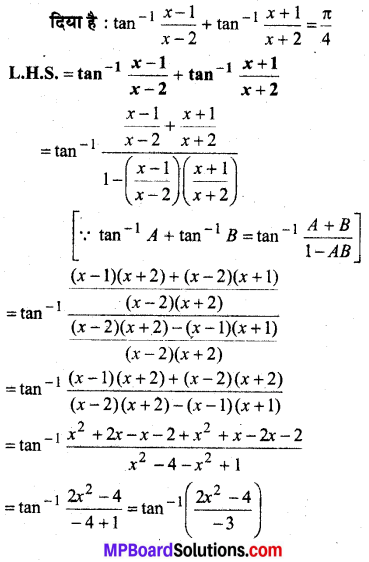
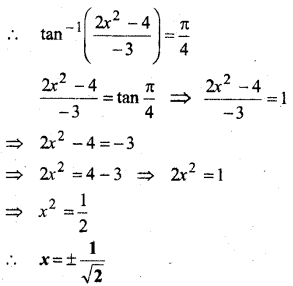
प्रश्न संख्या 16 से 18 में दिए गए प्रत्येक व्यंजक का मान ज्ञात कीजिए-
प्रश्न 16.
sin-1 (sin\(\frac{2 \pi}{3}\))
हल:
माना y = sin-1 (sin\(\frac{2 \pi}{3}\)), तब

![]()
प्रश्न 17.
tan-1 (tan\(\frac{3 \pi}{4}\))
हल:
माना y = tan-1 (tan\(\frac{3 \pi}{4}\))

प्रश्न 18.
tan (sin-1\(\frac{3}{5}\) + cot-1\(\frac{3}{2}\))
हल:
tan (sin-1\(\frac{3}{5}\) + cot-1\(\frac{3}{2}\))
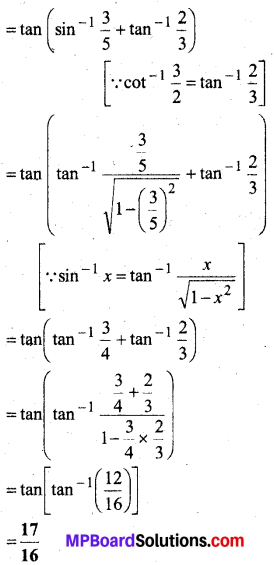
प्रश्न 19.
cos-1(cos \(\frac{7 \pi}{6}\)) का मान बराबर है-

हल:
cos-1[cos \(\frac{7 \pi}{6}\)]
cos-1 की मुख्य मान शाखा [0, π] है।

अत: विकल्प (B) सही है।
प्रश्न 20.
sin [\(\frac{\pi}{3}\) – sin-1(\(-\frac{1}{2}\))] का मान है-

हल:
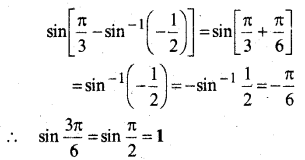
अत: विकल्प (D) सही है।
![]()
प्रश्न 21.
tan-1\( \sqrt{{3}} \) – cot-1 (-\( \sqrt{{3}} \)) का मान है
(A) π
(B) \(-\frac{\pi}{2}\)
(C) 0
(D) 2\( \sqrt{{3}} \)
हल:
