MP Board Class 9th Maths Solutions Chapter 14 Statistics Ex 14.4
Question 1.
The following number of goals were scored by a team in a series of 10 matches:
2, 3, 4, 5, 0, 1, 3, 3, 4, 3
Find the mean, median and mode of these scores.
Solution:
1.
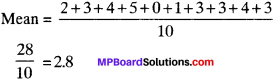
2. Arranging the data in ascending order, we get
0, 1, 2, 3, 3, 3, 3, 4, 4, 5
n = 10 (even)

3. As 3 is repeated maximum number of times i. e., 4 times.
∴ Mode = 3
Question 2.
In a mathematics test given to 15 students, the following marks (out of 100) are recorded:
41, 39, 48, 52, 46, 62, 54, 40, 96, 52, 98, 40, 42, 52, 60
Find the mean, median and mode of this data.
Solution:
1. Mean

2. Arranging the data in ascending order, we get
39, 40, 40, 41, 42, 46, 48, 52, 52, 52, 54, 60, 62, 96, 98
n = 15 (odd)
Median = (\(\frac{n+1}{2}\))th obseravation
= (\(\frac{15+1}{2}\))th observation = 8th obseravation
= 52.
3. As 52 is repeated maximum number of times i.e., 3 times
∴ Mode = 52
![]()
Question 3.
The following observations have been arranged in ascending order. If the median of the data is 63, find the value of x.
29, 32, 48, 50, x, x + 2, 72, 78, 84, 95
Solution:
Median = 63 (given)
n = 10 (even)

2 x 63 = 2x + 2
⇒ 126 = 2x + 2
⇒ 126 – 2 = 2x
⇒ 7x = 124
x = 62
Question 4.
Find the mode of 14, 25, 14, 28, 18, 17, 18, 14, 23, 22, 14, 18.
Solution:
As 14 repeated maximum number of times, mode =14.
Question 5.
Find the mean salary of 60 workers of a factory from the following table:
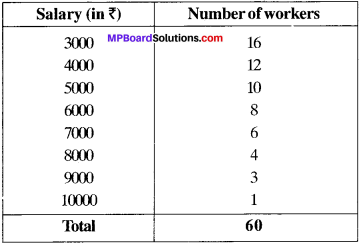
Solution:
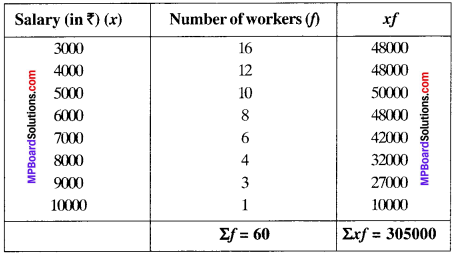
Question 6.
Give one example of a situation in which
- The mean is an appropriate measure of central tendency.
- The mean is not an appropriate measure of central tendency but the median is an appropriate measure of central tendency.
Solution:
1. Mean is a quantitative central tendency of a data.
Example:
For measuring central tendency of marks of a test we find the mean of the data.
2. Median is a qualitative central tendency of a data.
Example:
For measuring central tendency of beauty of a group of women, we determine the median of the data.